Dish or Parabola

A number of sources have been used to gain information for this page - including the excellent online microwave antenna book by Peter Wade [http:www.qsl.net/n1bwt/preface.htm W1GHZ]. The diagrams are original.

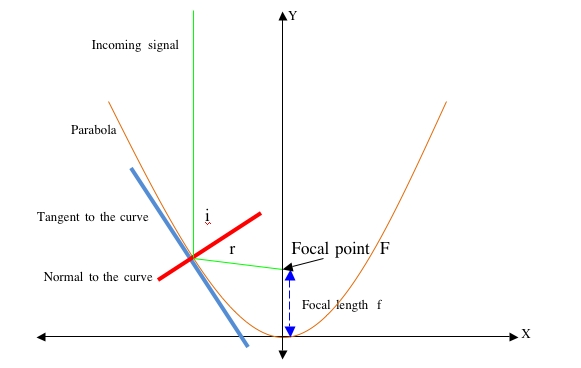
Parabolic Geometry

A Parabola is one of the “conic sections” and is defined as the locus (path) of a point that travels so that it is equidistant from a fixed point and a straight line. Algebraically this can be reduced to:

<math> y = ax^2</math> where a is a constant

More specifically, <math> y = \frac{x^2}{4f} </math> where f is the focal length – distance from the curve to the focal point

In the diagram above:

• the Y axis is central to the curve
• the tangent is a line that touches the curve at one point and has the same gradient as the curve at that point
• the normal is perpendicular to the tangent at the point of contact with the curve
• i is the angle of incidence – the angle between the incoming signal and the normal
• r is the angle of reflection – the angle between the reflected signal and the normal
• i = r Angle of incidence = Angle of reflection
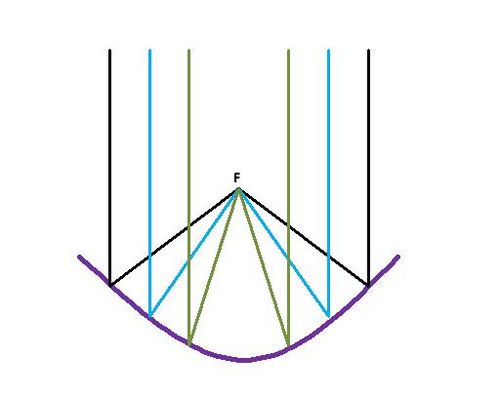
• a broad beam entering the parabola will be reflected to and concentrated at the focal point

During receive, the signal is concentrated at the focal point F. During transmit, a feed point at F will produce a beam of RF energy.

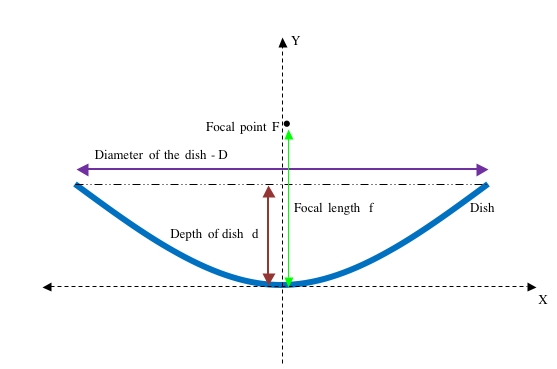
Finding the focal length of a parabolic dish

<math> f = \frac {D^2}{16d} </math> where D is the diameter of the dish and d is the depth of the dish

Gain of a dish antenna

Related wiki page - Gain

the gain of a dish antenna (compared to an isotropic standard) can be calculated thus:

<math> Gdbi = 10\times \mbox{log} \left( \eta \frac {4 \pi}{\lambda^2}A \right) </math> where:

<math> \eta </math> is the efficiency of the dish

<math> A </math> is the area of the dish

<math> \lambda </math> is the wavelength of the signal

The efficiency is perhaps the most difficult variable to maximise - generally 50% is considered to be reasonable, but 70% - 80% can be achieved with very good feeds.

For practical purposes, the most critical measurement affecting the gain (regardless of dish size) of a dish antenna has been found to be placing the feedpoint exactly at the focal length f. Paul Wade - W1GHZ, gives the following relative gain measurements for a 22inch dish with <math> \frac{f}{D} =0.39 </math> operating at 10GHz:

Feed distance	Relative gain
8.125"	-0.6dB
8.250"	0dB
8.375"	-0.3dB
8.625"	-1.7dB